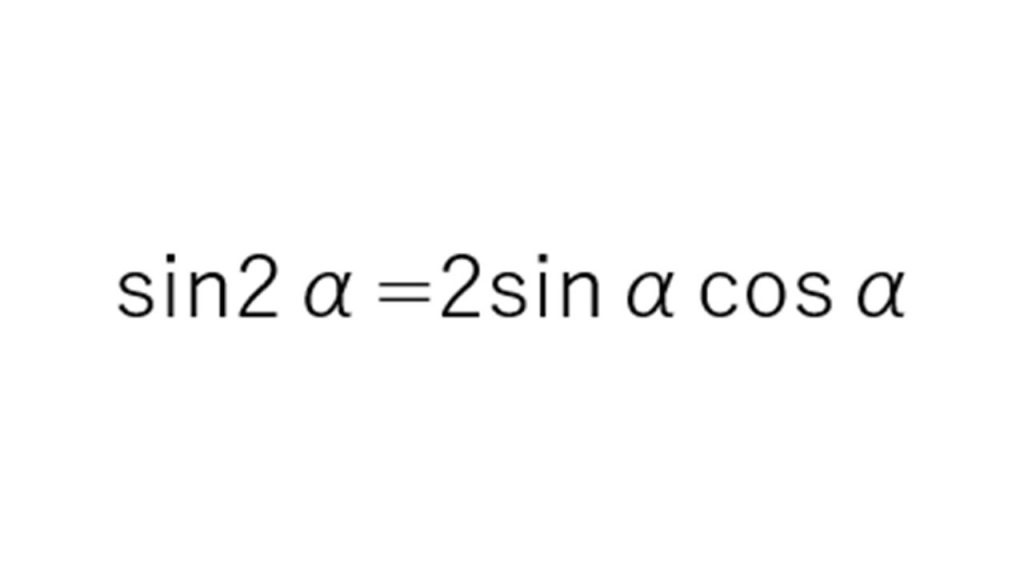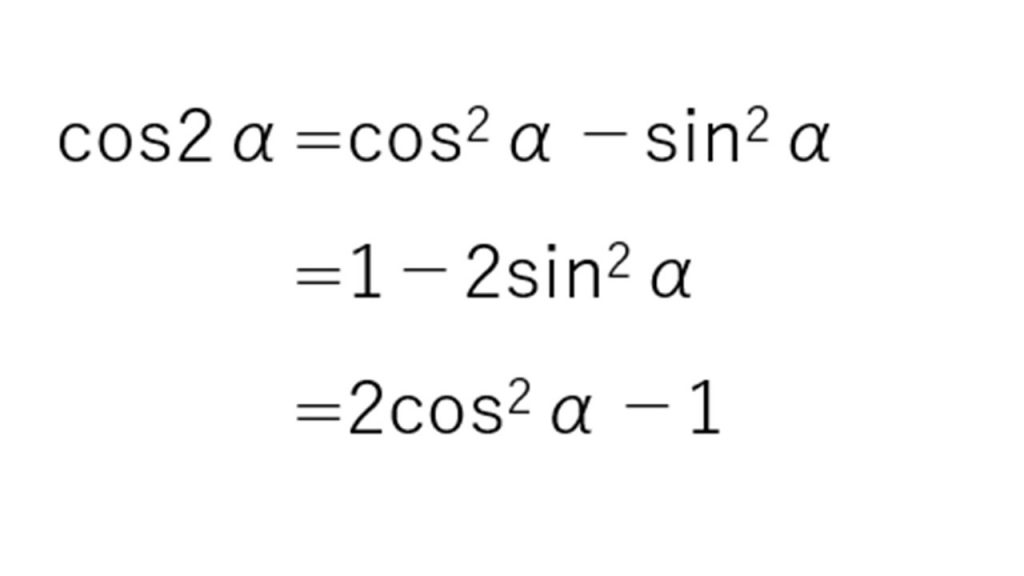(1)例題
(例題作成中)
(2)例題の答案
(答案作成中)
(3)解法のポイント
3倍角の公式は覚える必要はありません(もちろん覚えるに越したことはありませんが)
加法定理と2倍角の公式を使うと3倍角を求めることができます。以下のその方法を示します。
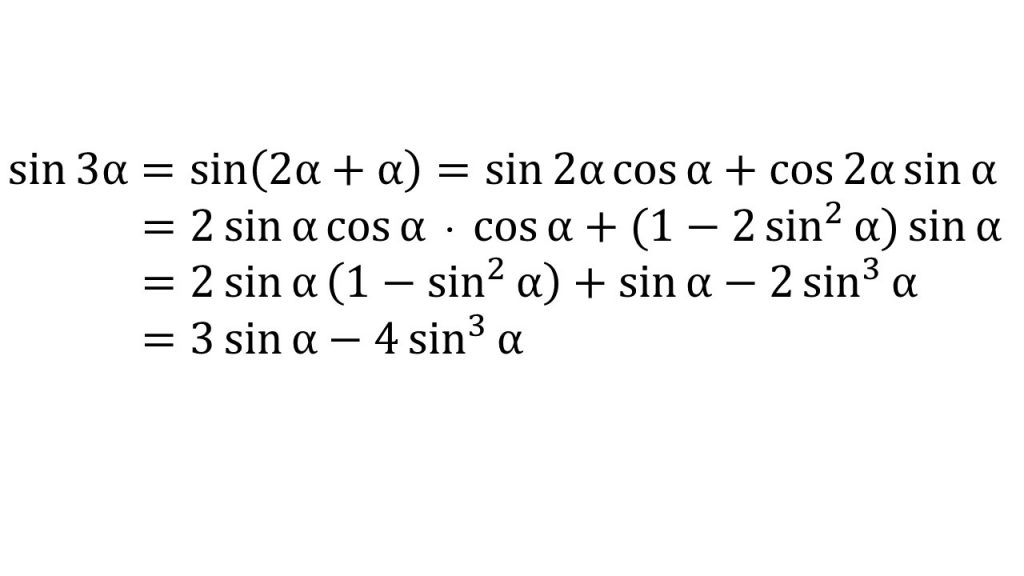
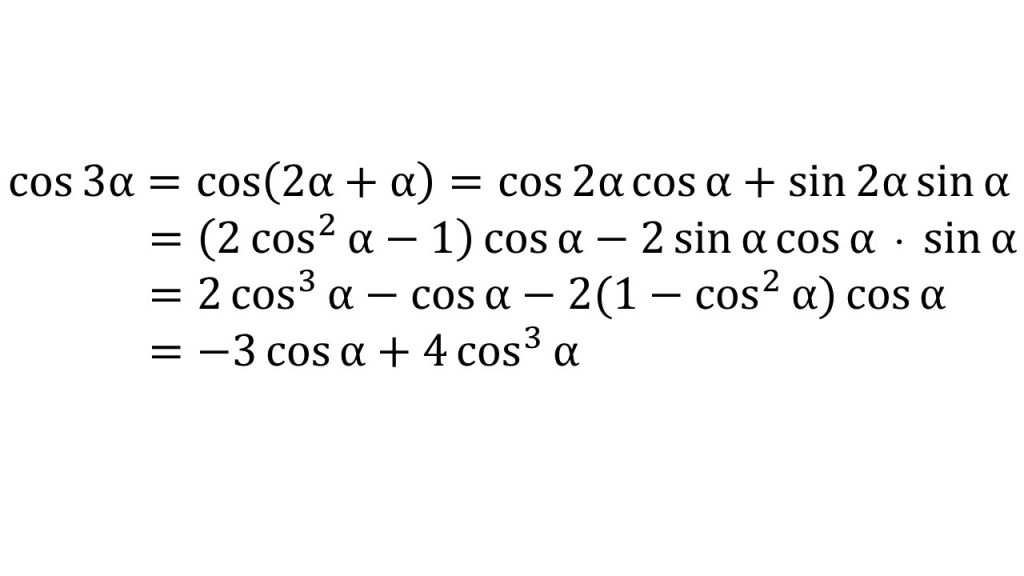
(4)必要な知識
①加法定理
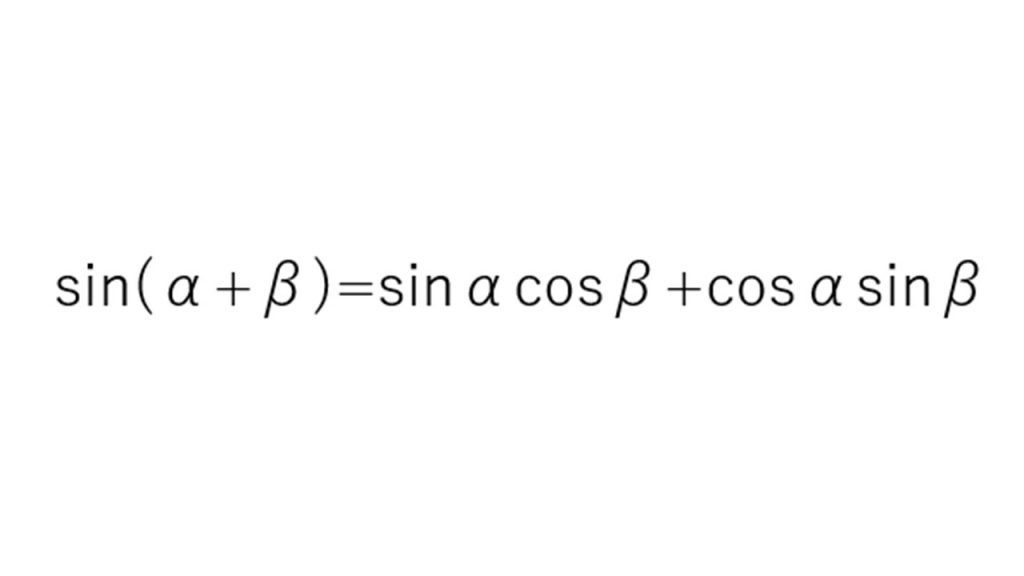
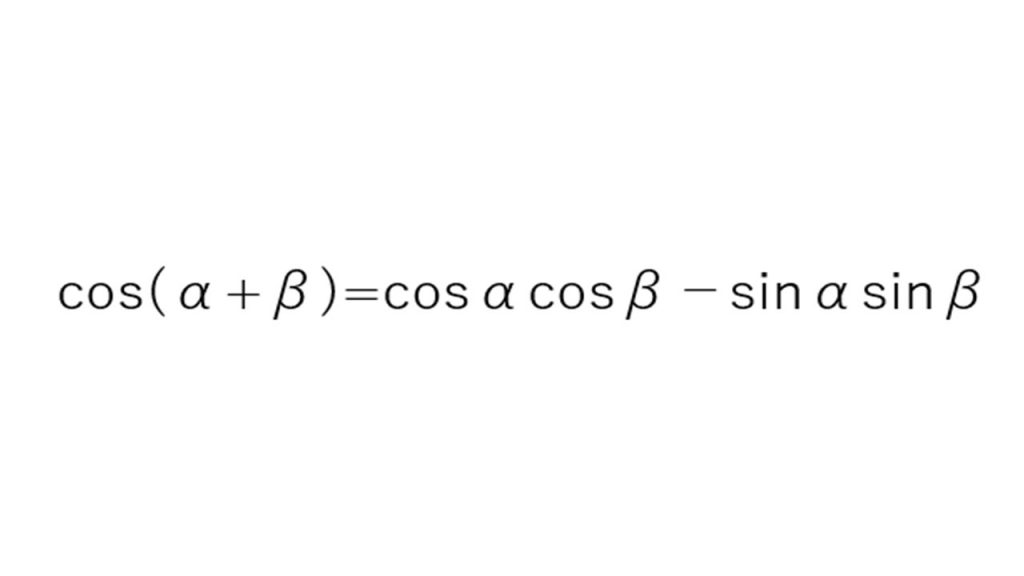
②2倍角の公式
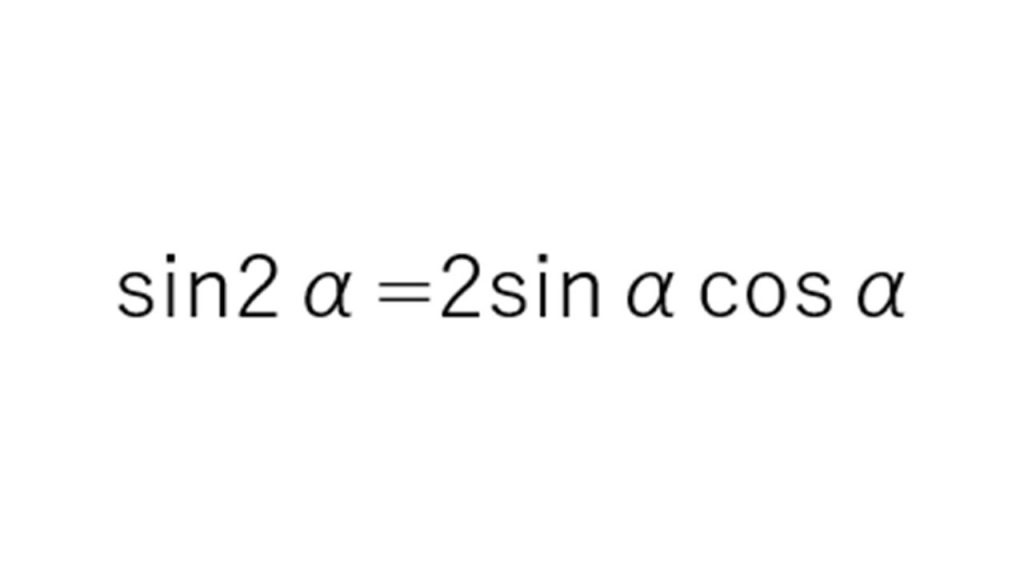
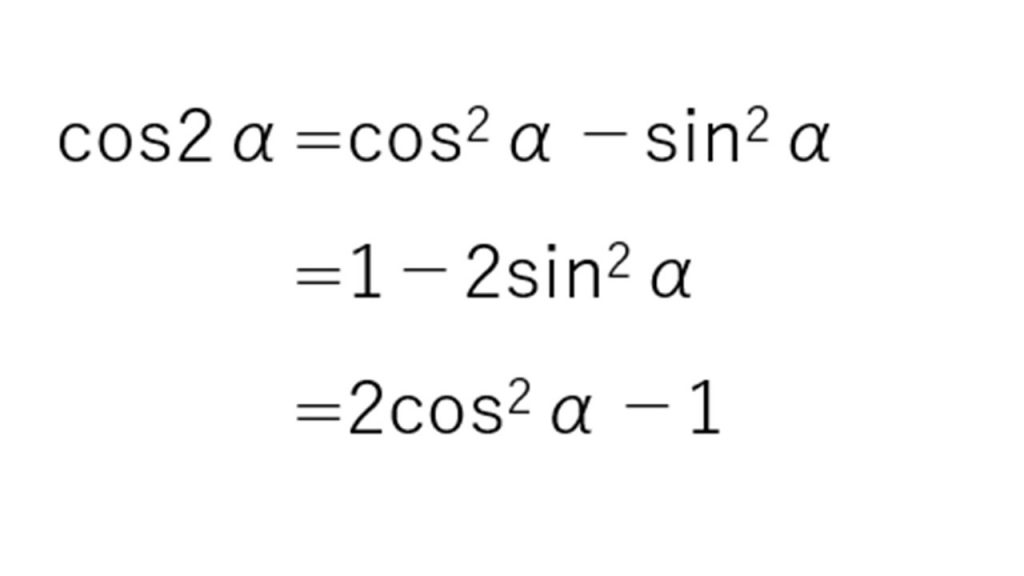
(1)例題
(例題作成中)
(2)例題の答案
(答案作成中)
(3)解法のポイント
3倍角の公式は覚える必要はありません(もちろん覚えるに越したことはありませんが)
加法定理と2倍角の公式を使うと3倍角を求めることができます。以下のその方法を示します。
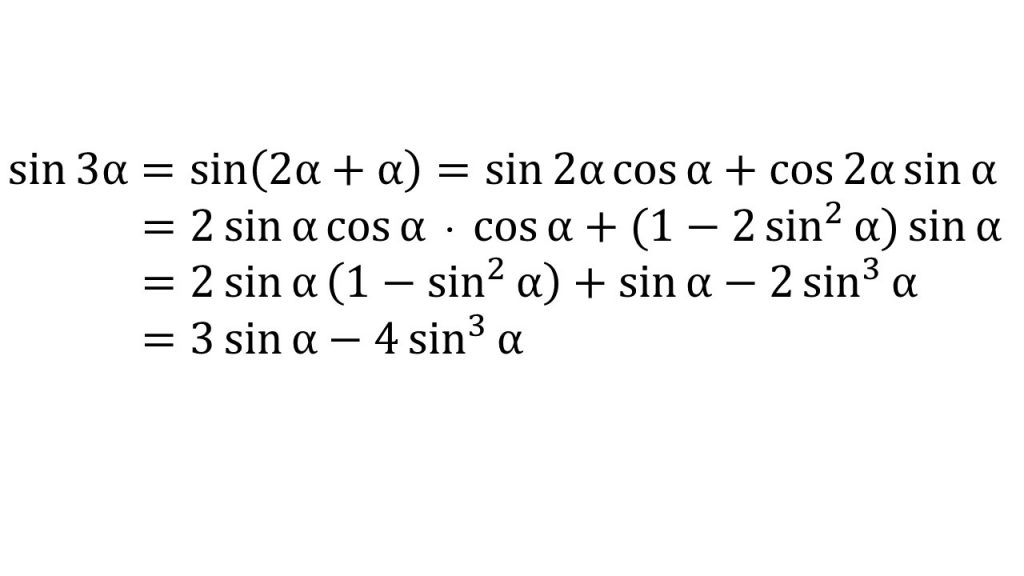
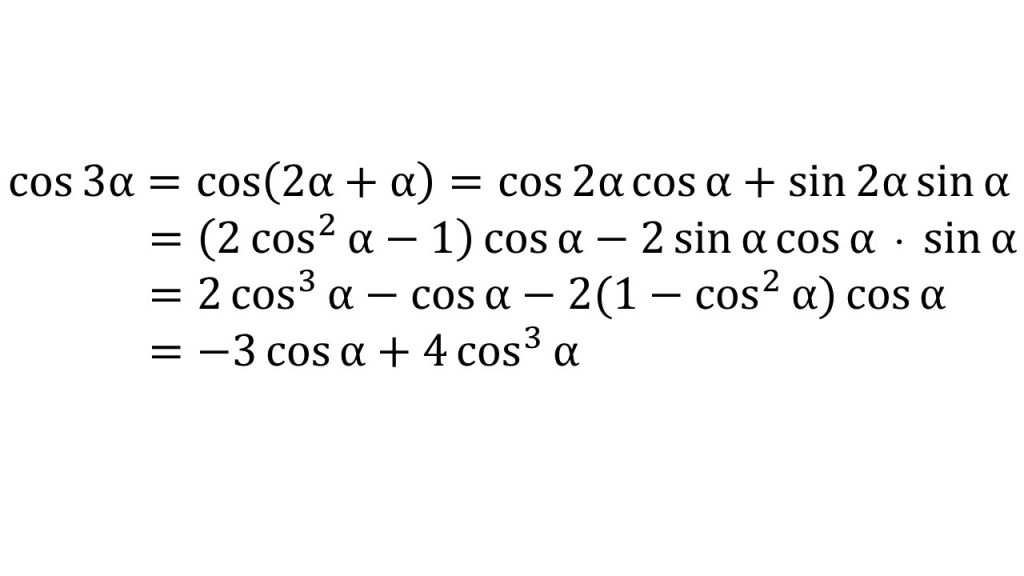
(4)必要な知識
①加法定理
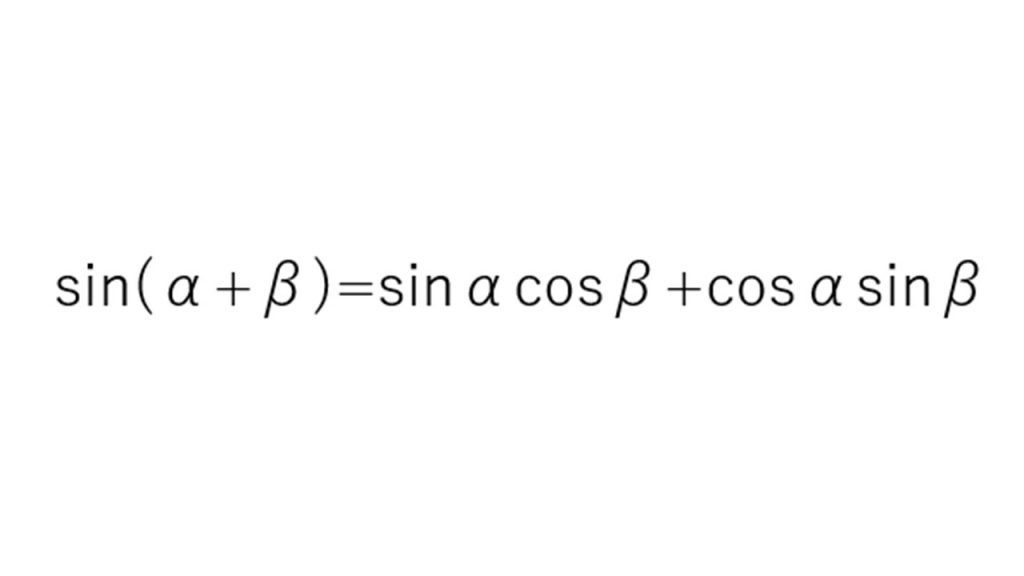
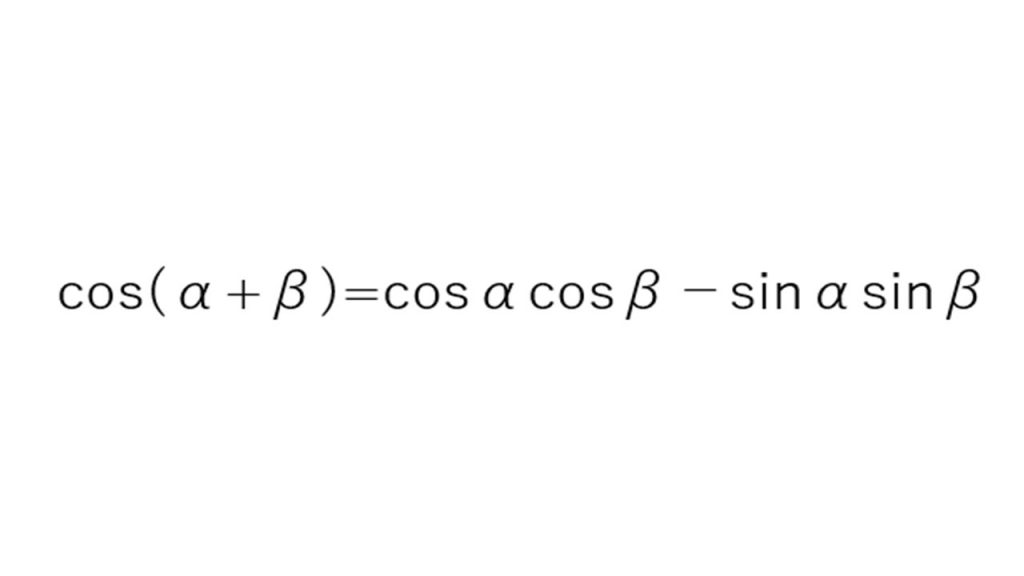
②2倍角の公式